Question

The graph of a line goes through the points (-4,3) and (6,8). What is the equation of the line in slope-intercept form?

Enter the correct answer in the box by replacing m and b with the appropriate values.
음 (0) 미
Of
+
a, sin cos tan sin costan!
μ ρ Φ
CSC seccot log log. In
ΖΔ
Σ
U (0)
X
5
A
>
S
2
II
1
y = mx + b
I
Submit
Reset
Type here to search
73°F Mostly cloudy
327 PM
7/3/2001

Answer 1

`y=(1)/(2)x+5`

Explanation:

Hi there!

Slope intercept form:
`y=mx+b` where m is the slope and b is the y-intercept (the value of y when x is 0)

1) Determine the slope (m)

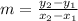`m=(y_2-y_1)/(x_2-x_1)` where two points that lie on the line are
`(x_1,y_1)` and
`(x_2,y_2)`

Plug the given points (-4,3) and (6,8) into the equation

`m=(8-3)/(6-(-4))\\m=(8-3)/(6+4)\\m=(5)/(10)\\m=(1)/(2)`

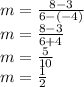
Therefore, the slope of the line is
`(1)/(2)`. Plug this into
`y=mx+b` :

`y=(1)/(2)x+b`

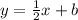
2) Determine the y-intercept (b)

`y=(1)/(2)x+b`

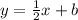
Plug in one of the given points and solve for b

`8=(1)/(2)(6)+b\\8=3+b`

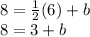
Subtract 3 from both sides to isolate b

`8-3=3+b-3\\5=b`

Therefore, the y-intercept is 5. Plug this back into
`y=(1)/(2)x+b`:

`y=(1)/(2)x+5`

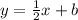I hope this helps!