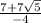Question

How would the fraction
`(7)/(1-√(5) )` be rewritten if its denominator is rationalized using difference of squares?

Answer 1

`(7 + 7 √(5) )/( - 4)`

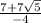
Explanation:

We would multiply the fraction by its conjugate

( A conjugate is a expression that has the same integer or number values but have different signs) for example

`5x + 2`

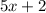
and

`5x - 2`

ARE Conjugates.

The conjugate of

`1 - √(5)`

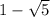
is

`1 + √(5)`

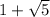
So this means we will multiply the expression by 1 plus sqr root of 5 on the numerator and denominator.

Our new numerator will be

`7 * (1 + √(5) ) = 7 + 7 √(5)`

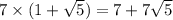
We can apply the difference of squares for the denominator.

`(x + y)(x - y) = x {}^(2) - {y}^(2)`

So our denominator will be

`1 - 5 = - 4`

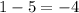
So our rationalized fraction will be

`(7 + 7 √(5) )/( - 4)`