Answer:
13.94 rpm
Step-by-step explanation:
Given that,
The diameter of the pulley, d = 14 foot
Radius, r = 7 foot
The linear velocity of the pulley, v = 14 mph = 20.53 ft/s
We need to find the angular velocity in rpm.
We know that, the relation between the linear velocity and the angular velocity is as follows :
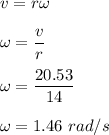
or
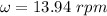
So, the angular velocity of the pulley is 13.94 rpm.