Question

A parabola can be drawn given a focus of (-9, -7) and a directrix of x = 9. Write

the equation of the parabola in any form.

Answer 1

Check the picture below, so the parabola looks more or less like so, with a vertex at (0 , -7), let's recall the vertex is half-way between the focus point and the directrix.

so this horizontal parabola opens up to the left-hand-side, meaning that the "P" distance is a negative value.

`\textit{horizontal parabola vertex form with focus point distance} \\\\ 4p(x- h)=(y- k)^2 \qquad \begin{cases} \stackrel{vertex}{(h,k)}\qquad \stackrel{focus~point}{(h+p,k)}\qquad \stackrel{directrix}{x=h-p}\\\\ p=\textit{distance from vertex to }\\ \qquad \textit{ focus or directrix}\\\\ \stackrel{`

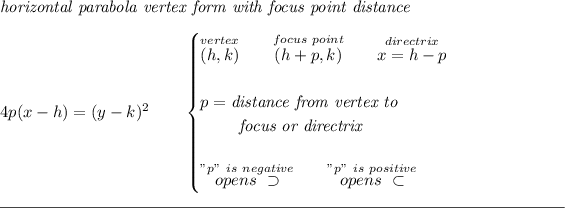
`\begin{cases} h=0\\ k=-7\\ p=-9 \end{cases}\implies 4(-9)(x-0)~~ = ~~[y-(-7)]^2 \\\\\\ -36x=(y+7)^2\implies x=-\cfrac{1}{36}(y+7)^2`