Answer:
Explanation:
a). For function 'f',
Slope of a linear function passing through two points
 and
and
 is given by,
is given by,
Slope =
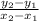
For the function 'f' given in the table,
Slope of the linear function passing through two points (-1, -5) and (0, -1) given in the table,
Slope =
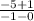
= 4
Equation of the line passing through a point (0, -1) and slope = 4 will be,
y - y' = m(x - x')
y + 1 = 4(x - 0)
y = 4x - 1
f(x) = 4x - 1
For function 'g',
Equation of the function 'g' has been given as,
g(x) = 4x + 3
By comparing this equation with the slope-intercept equation of a line,
y = mx + b
Therefore, slope of the function 'g' is,
m = 4
Since slopes of both the functions are same, linear graphs of both the functions will be parallel.
b). Equation of the function 'f' is,
f(x) = 4x - 1
y-intercept of the function = -1
Equation of function 'g',
g(x) = 4x + 3
y-intercept = 3
Therefore, function 'g' will have the greater y-intercept.