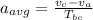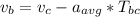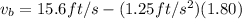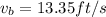Answer:
a)
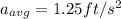
b)
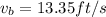
Step-by-step explanation:
From the question we are told that:
Speed at point A
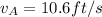
Speed at point C
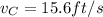
Time from Point A to C

Time from Point B to C

Generally the equation for acceleration From A to B is mathematically given by
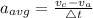

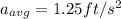
Generally the equation for cart speed at B is mathematically given by