Question

Exercise 2.2.3: The cardinality of a power set. (a) What is the cardinality of P({1, 2, 3, 4, 5, 6})

Answer 1

Cardinality of the power set of the given set =
`2^6=64`

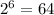
Explanation:

Power set is the set of all the possible subsets that can be formed from the given set including the null set and the set itself.

Example set:

{1,2,3}

All the possible subsets of this set:

{}; {1}; {2}; {3}; {1,2,3}; {1,2}; {1,3}; {2,3}

The power set of the above set is written as:

P({ {}, {1}, {2}, {3}, {1,2}, {1,3}, {2,3}, {1,2,3} })

Since the no. of elements in the above power set in this example is 8 therefore its cardinality is 8.

Cardinality of the power set of a given set is expressed by a formula:
`2^n`

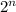
where n is the cardinality (no. of elements) of the given set whose power set is to be formed for determining cardinality of the power set.

Hence in the given case, we have n = 6.