Answer:
The designed life should be of 21,840 vehicle miles.
Explanation:
Normal Probability Distribution
Problems of normal distributions can be solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the z-score of a measure X is given by:
, the z-score of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Mean of 35,000 vehicle miles and a standard deviation of 7,000 vehicle miles.
This means that
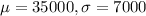
Find its designed life if a .97 reliability is desired.
The designed life should be the 100 - 97 = 3rd percentile(we want only 3% of the vehicles to fail within this time), which is X when Z has a p-value of 0.03, so X when Z = -1.88.

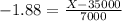
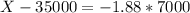

The designed life should be of 21,840 vehicle miles.