Question

We have 9 pens, of which 5 are green ink, 3 are red ink, and 1 is black. If we put the pens in a line, how many arrangements are possible

Answer 1

504 arrangements are possible

Explanation:

Arrangements of n elements:

The number of arrangements of n elements is given by:

`A_(n) = n!`

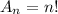
Arrangements of n elements, divided into groups:

The number of arrangements of n elements, divided into groups of
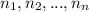`n_1, n_2,...,n_n` elements is given by:

`A_(n)^(n_1,n_2,...,n_n) = (n!)/(n_1!n_2!...n_n!)`

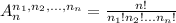
In this case:

9 pens, into groups of 5, 3 and 1. So

`A_(9)^(5,3,1) = (9!)/(5!3!1!) = 504`

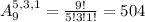
504 arrangements are possible