Answer: Choice A. (2,2), (3,1), (4,2)
==========================================================
Step-by-step explanation:
The graph of
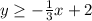 has the boundary y = (-1/3)x+2 which is a solid line. This line goes through (0,2) and (3,1). We shade above the boundary because of the "greater than" sign.
has the boundary y = (-1/3)x+2 which is a solid line. This line goes through (0,2) and (3,1). We shade above the boundary because of the "greater than" sign.
The graph of y < 2x+3 has a dashed boundary line of y = 2x+3, and we shade below the boundary because of the "less than" sign.
The two regions overlap in the upper right corner where it's shaded in the darkest color. The points (2,2), (3,1) and (4,2) are in this upper right corner region. If we plug the coordinates of each point into each inequality, then we'll get true statements.
For instance, let's try (x,y) = (2,2) into the first inequality

Which is true since 2 is indeed larger than 1.33, so that confirms (2,2) is in the shaded region for
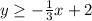
Let's check the other inequality as well
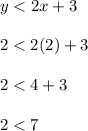
That works too. So (2,2) is in BOTH shaded regions at the same time; hence, it's a solution to the system. You should find that (3,1) and (4,2) work for both inequalities also. This will confirm choice A is the answer.
--------------------------------
Extra info (optional section)
A point like (3,-1) does not work for the first inequality as shown below

Since -1 is neither equal to 1, nor is -1 larger than 1 either. The false statement at the end indicates (3,-1) is not a solution to that inequality.
Based on the graph, the point (3,-1) is not above the blue solid boundary line. All of this means we can rule out choice B.
You should find that (1,-2) is a similar story, so we can rule out choice C. Choice D can be ruled out because (2,0) is not a solution to the first inequality.