Answer:
The sample is about 34380 years old.
Step-by-step explanation:
The amount of Carbon-14 mass diminishes exponentially in time, whose model is described below:
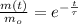 (1)
(1)
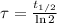 (2)
(2)
Where:
 - Initial mass, in grams.
- Initial mass, in grams.
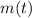 - Current mass, in grams.
- Current mass, in grams.
 - Time, in years.
- Time, in years.
 - Time constant, in years.
- Time constant, in years.
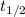 - Half-life, in years.
- Half-life, in years.
If we know that
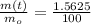 and
and
 , then the age of the sample is:
, then the age of the sample is:
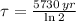
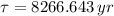
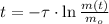
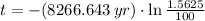
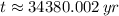
The sample is about 34380 years old.