Answer:
the distance is 16
Explanation:
Hi there!
We are given point A (-4,-13) and point B (-4,3). We need to find the distance between those two points
the distance formula is given as
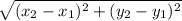 where (
where (
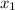 ,
,
 ) and (
) and (
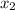 ,
,
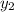 ) are points
) are points
we are given 2 points, which is what we need for the formula. However, let's label the values of the points to avoid any confusion
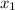 =-4
=-4
 =-13
=-13
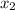 =-4
=-4
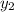 =3
=3
now substitute those values into the formula. Remember: the formula uses SUBTRACTION.
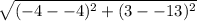
simplify
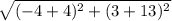
now add the values inside the parenthesis that are under the radical
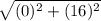
raise everything under the radical to the second power

add under the radical
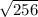
now take the square root of 256
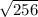 =16
=16
so the distance between point A and point B is 16
Hope this helps! :)