Answer:
0.8743 = 87.43% probability that more than one accident occurs per year
Explanation:
In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

In which
x is the number of sucesses
e = 2.71828 is the Euler number
 is the mean in the given interval.
is the mean in the given interval.
Buchtal, a manufacturer of ceramic tiles, reports on average 3.1 job-related accidents per year.
This means that
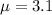
What is the probability that more than one accident occurs per year?
This is:
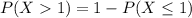
In which
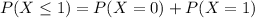
Then

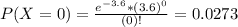
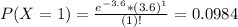
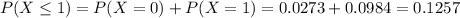
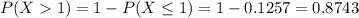
0.8743 = 87.43% probability that more than one accident occurs per year