Question

Consider a tall building of height 200.0 m. A stone A is dropped from the top (from the cornice of the building). One second later another stone B is thrown vertically up from the point on the ground just below the point from where stone A is dropped.Birthstones meet at half the height of the tower. (a) Find the initial velocity of vertical throw of stone B.(b) Find the velocities of A and B, just before they meet.

Answer 1

a) v₀ = 44.27 m / s, b) stone A v = 44.276 m / s, stone B v = 0.006 m / s

Step-by-step explanation:

a) This is a kinematics exercise, let's start by finding the time it takes for stone A to reach half the height of the building y = 100 m

y = y₀ + v₀ t - ½ gt²

as the stone is released its initial velocity is zero

y- y₀ = 0 - ½ g t²

t =
`√( -2(y-y_o)/g)`

t =
`√( -2(100-200)/9.8)`

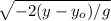
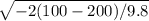
t = 4.518 s

now we can find the initial velocity of stone B to reach this height at the same time

y = y₀ + v₀ t - ½ g t²

stone B leaves the floor so its initial height is zero

100 = 0 + v₀ 4.518 - ½ 9.8 4.518²

100 = 4.518 v₀ - 100.02

v₀ =
`(100-100.02)/(4.518)`

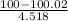
v₀ = 44.27 m / s

b) the speed of the two stones at the meeting point

stone A

v = v₀ - gt

v = 0 - 9.8 4.518

v = 44.276 m / s

stone B

v = v₀ -g t

v = 44.27 - 9.8 4.518

v = 0.006 m / s