Answer:
The perimeter is minimum for Length and width both are
 .
.
Explanation:
Area, A = 3 square metre
Let the length is L and width is W.
Area = L W
3 = L W.....(1)
The perimeter is given by
P = 2 (L + W)
Substitute the value of from (1)
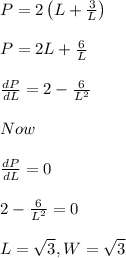
Now
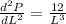
It is alays positive, so the perimeter is minimum.