Answer:
Suppose that we have a given function f(x)
The average rate of change of the function between two values x₁ and x₂ is given by:
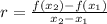
a) We want to find the average (rate) of change on the size of population from 1985 to 1995.
We have that:
f(1985) = 750
f(1995) = 1500
Then we have:
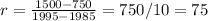
This means that the population of trees increases, in average, at a rate of 75 trees per year.
b) What is the density of trees each year that they were counted?
This will be equal to the quotient between the number of trees and the area.
1985: number of trees = 750 pines
area = 250 ha
Then the density is:
D(1985) = (750 pines)/(250 ha) = 3 pines/ha
So 1985, there were 3 pines per hectare.
1990: number of trees = 1250 pines
area = 250 ha
Then the density is:
D(1990) = (1250 pines)/(250 ha) = 5 pines/ha
1995: number of trees = 1500 pines
area = 250 ha
The density is:
D(1995) = (1500 pines)/(250 ha) = 6 pines/ha
3) now we want to get the average change between 1985 and 1995 in the density, this will be:

So, on average, each year the number of pines per hectare increases by 0.3