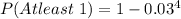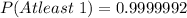Answer:
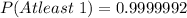
Explanation:
Given
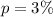 --- rate of hard disk drives failure
--- rate of hard disk drives failure
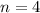 --- number of hard disk drives
--- number of hard disk drives
See comment for complete question
Required
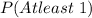
First, calculate the probability that the none of the 4 selected is working;
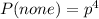
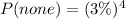
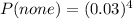
Using the complement rule, the probability that at least 1 is working is:

This gives: