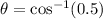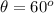Question

Find the angle between the vectors ????=????+???? and ????=−????+????. (Give an exact answer. Use symbolic notation and fractions where needed.)

Answer 1

The angle between them is 60 degrees

Explanation:

Given

`a = 2i + j -3k`

`b = 3i - 2j -k`

Required

The angle between them

The cosine of the angle between them is:

`\cos(\theta) = (a\cdot b)/(|a|\cdot |b|)`

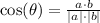
First, calculate a.b

`a \cdot b =(2i + j -3k) \cdot (3i - 2j -k)`

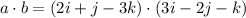
Multiply the coefficients of like terms

`a \cdot b =2 * 3 - 1 * 2 - 3 * -1`

`a \cdot b =7`

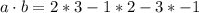
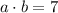
Next, calculate |a| and |b|

`|a| = \sqrt{2^2 + 1^2 + (-3)^2`

`|a| = \sqrt{14`

`|b| = √(3^2 + (-2)^2 + (-1)^2)`

`|b| = √(14)`

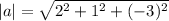
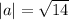
Recall that:

`\cos(\theta) = (a\cdot b)/(|a|\cdot |b|)`

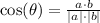
This gives:

`\cos(\theta) = (7)/(√(14) * √(14))`

`\cos(\theta) = (7)/(14)`

`\cos(\theta) = 0.5`

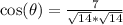
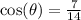
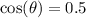
Take arccos of both sides

`\theta =\cos^(-1)(0.5)`

`\theta =60^o`