Answer: Choice D
The graph will be discrete because there is no such thing as a partial person to sign up, and the booth is set up once each day for sign ups.
=========================================================
Step-by-step explanation:
Let's start with the independent variable d. This acts as the variable x. It's the input. The value of d only takes on positive whole numbers (eg: d = 1, d = 2, d = 3, etc). We cannot have something like d = 2.718
So this bit of evidence shows that our function is discrete. Discrete input values (d) plug into the function to produce corresponding discrete output values (m).
Furthermore, we know that m is discrete because the number of people cannot be a fractional or decimal number. We can't have half a person for instance.
---------------
A quick way to see if a set is discrete or continuous is to ask the question: "is it possible to apply the midpoint formula for ANY two values, and have the output make sense?"
So a set like {1,2,3,4,5,...} is discrete because the midpoint of 2 and 3 is 2.5, but that value is not in the set mentioned.
In other words, discrete sets have "gaps" so to speak, while continuous ones do not.
---------------
Another useful property is that let's say that a < x < b, and x is drawn from the domain set. This reduced set will be finite if we're dealing with discrete data. Eg: The set {1,2,3,4,5,...} has the subset {2,3,4} which is finite and discrete.
In contrast, the subset of real numbers x such that
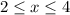 is continuous and this subset is infinitely large (has infinitely many members) because we could have things like 2.718 or 3.14 etc
is continuous and this subset is infinitely large (has infinitely many members) because we could have things like 2.718 or 3.14 etc