Question

Which ordered pairs are in the solution set of the system of linear inequalities?

y > Negative one-thirdx + 2

y < 2x + 3

On a coordinate plane, 2 straight lines are shown. The first solid line has a negative slope and goes through (0, 2) and (6, 0. Everything to the right of the line is shaded. The second dashed line has a positive slope and goes through (negative 3, negative 3) and (0, 3). Everything above the line is shaded.

Answer 1

A. (2, 2), (3, 1), (4, 2)

Explanation:

Answer 2

Options:

(2, 2), (3, 1), (4, 2)

(2, 2), (3, –1), (4, 1)

(2, 2), (1, –2), (0, 2)

(2, 2), (1, 2), (2, 0)

Answer:

A. (2, 2), (3, 1), (4, 2)

Explanation:

Given

`y > -(1)/(3)x + 2`

`y < 2x + 3`

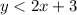
Required

Solve for x and y

To solve this, we make use of graphical method (see attachment for graph)

All points that lie on the shaded region are true for the inequality

Next, we plot each of the given options on the graph

A. (2, 2), (3, 1), (4, 2)

All 3 points lie on the shaded region.

Hence, (a) is true