Question

A company that manufactures hair ribbons knows that the number of ribbons it can sell each week, x, is related to the price p per ribbon by the equation below.

x = 1,000 − 100p
At what price should the company sell the ribbons if it wants the weekly revenue to be $1,600? (Remember: The equation for revenue is R = xp.)
p = $ (smaller value)
p = $ (larger value)

Answer 1

Given:

The number of ribbons it can sell each week, x, is related to the price p per ribbon by the equation:

`x=1000-100p`

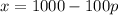
To find:

The selling price if the company wants the weekly revenue to be $1,600.

Solution:

We know that the revenue is the product of quantity and price.

`R=xp`

`R=(1000-100p)p`

`R=1000p-100p^2`

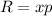
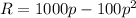
We need to find the value of p when the value of R is $1600.

`1600=1000p-100p^2`

`1600-1000p+100p^2=0`

`100(16-10p+p^2)=0`

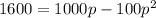
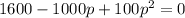
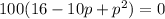
Divide both sides by 100.

`p^2-10p+16=0`

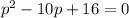
Splitting the middle term, we get

`p^2-8p-2p+16=0`

`p(p-8)-2(p-8)=0`

`(p-8)(p-2)=0`

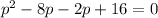
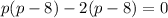
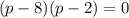
Using zero product property, we get

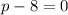`p-8=0` or
`p-2=0`

`p=8` or
`p=2`

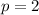
Therefore, the smaller value of p is $2 and the larger value of p is $8.