Answer:
(
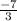 ,
,
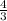 )
)
Explanation:
Hi there!
We are given the following system of equations:
-3x-3y=3
-5x+y=13
and we need to find the solution (the point at which the 2 lines intersect)
let's solve this by substitution, where we will set one variable equal to an expression containing the other variable, and then substitute that expression into the other equation to solve for the variable that the expression from earlier contains, and then use the value of the solved variable to find the value of the first variable
in the second equation, add 5x to both sides to isolate y by itself
y=5x+13
now substitute 5x+13 as y in -3x-3y=3
-3x-3(5x+13)=3
do the distributive property
-3x-15x-39=3
combine like terms
-18x-39=3
add 39 to both sides
-18x=42
divide both sides by -18
x=
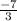
now we need to find y
remember: y=5x+13
substitute
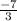 as x in y=5x+13
as x in y=5x+13
y=5(
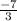 )+13
)+13
multiply
y=
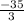 +13
+13
add
y=
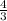
So the answer is x=
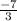 , y=
, y=
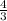 . As a point, it's (
. As a point, it's (
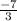 ,
,
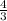 )
)
Hope this helps! :)