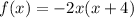Answer:
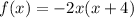
Explanation:
We want to find the equation of a quadratic function in factored form with zeros at x = -4 and x = 0 that passes through the point (-3, 6).
The factored form of a quadratic is given by:
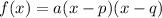
Where p and q are the zeros and a is the leading coefficient.
Since we have zeros at x = -4 and x = 0, let p = -4 and q = 0. Substitute:
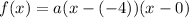
Simplify:
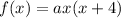
And since we know that the function passes through the point (-3, 6), f(x) = 6 when x = -3. Thus:
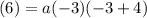
Simplify:
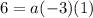
Thus:

So, our quadratic function is: