Question

Determine the type and number of solutions of −4x2 − 3x + 7 = 0.

two real solutions


two imaginary solutions


one real solution and one imaginary solution


one real solution

Answer 1

Explanation:

You have to use the discriminant for this. If the quadratic is
`-4x^2-3x+7`, then

a = -4, b = -3, and c = 7. The formula for finding the discriminant is

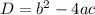`D=b^2-4ac` which comes from the quadratic formula, but without the square root sign. Filling in:

`D=(-3)^2-4(-4)(7)` which simplifies down to

D = 9 + 112 so

D = 121. This is a perfect square, so the solutions will be 2 real. Just so you know, you will NEVER have a solution like the one offered in the third choice down. If you have one imaginary root, you will ALWAYS have a second by the conjugate rule.