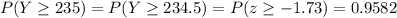Question

Retention rates in a weight loss program. Americans spend over $30 billion annually on a variety of weight loss products and services. In a study of retention rates of those using the Rewards Program at Jenny Craig in 2005, it was found that about 18% of those who began the program dropped out in the first four weeks.10 Assume we have a random sample of 300 people beginning the program.

a) What is the mean number of people who would drop out of the Rewards Program within four weeks in a sample of this size? What is the standard deviation?
b) What is the approximate probability that at least 235 people in the sample will still be in the Rewards Program after the first four weeks?

Answer 1

(a)

`\mu=54`

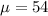
The standard deviation is

`\sigma=6.6543`

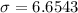
(b)

`\mu=246\\\sigma=6.6543`

Here sample size is large and np and n(1-p) are both greater than 30. So we can use a normal approximation of binomial distribution. z-score for Y = 234.5 (using continuity correction) is

`z=-1.73`

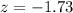
So the approximate probability that at least 235 people in the sample will still be in the Rewards Program after the first four weeks is

`P(Y\geq 235)=P(Y\geq 234.5)=P(z\geq -1.73)=0.9582`

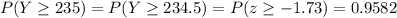
Explanation:

Let X is a random variable that shows the number of people who would drop out of the Rewards Program within four weeks. Here X has binomial distribution with parameters n = 300 and p = 0.18.

(a)

The mean number of people who would drop out of the Rewards Program within four weeks in a sample of this size is

`\mu=np=300\cdot 0.18=54`

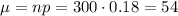
The standard deviation is

`\sigma=√(np(1-p))=√(300\cdot 0.18\cdot 0.82)=6.6543`

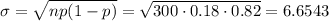
(b)

Let Y is a random variable that shows the number of people in the sample who will still be in the Rewards Program after the first four weeks. Here Y has a binomial distribution with parameters n= 300 and p=0.82. So mean of Y is

`\mu=np=300\cdot 0.82=246\\\sigma=√(np(1-p))=√(300\cdot 0.18\cdot 0.82)=6.6543`

Here sample size is large and np and n(1-p) are both greater than 30. So we can use a normal approximation of binomial distribution. z-score for Y = 234.5 (using continuity correction) is

`z=(Y-\mu)/(\sigma)=(234.5-246)/(6.6543)=-1.73`

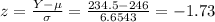
So the approximate probability that at least 235 people in the sample will still be in the Rewards Program after the first four weeks is

`P(Y\geq 235)=P(Y\geq 234.5)=P(z\geq -1.73)=0.9582`