Question

If x follows a normal distribution with a mean of 10 and

standard deviation of 3; find out the following
A) Find the value of x = xº such that P(x 5 xº) = 5%
B) Find the value of x = xº such that P(x 2 x°) = 1%

Answer 1

The answer is below

Step-by-step explanation:

The z score is used to determine by how many standard deviations the raw score is above or below the mean. The z score is given by:

`z=(x-\mu)/(\sigma) \\\\where\ x=raw\ score, \mu=mean,\ \sigma=standard\ deviation`

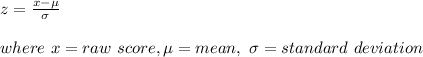
a) P(x ≥ x⁰) = P(z ≥ z⁰) = 1 - P(z < z⁰)

1 - P(z < z⁰) = 1%

P(z < z⁰) = 99%

z⁰ = 2.33

z° = (x° - μ)/σ

2.33 = (x° - 10)/3

x⁰ = 16.99

b) P(x ≥ x⁰) = P(z ≥ z⁰) = 1 - P(z < z⁰)

1 - P(z < z⁰) = 10%

P(z < z⁰) = 90%

z⁰ = 1.28

z° = (x° - μ)/σ

1.28 = (x° - 10)/3

x⁰ = 13.84

c) P(x ≥ x⁰) = P(z ≥ z⁰) = 1 - P(z < z⁰)

1 - P(z < z⁰) = 5%

P(z < z⁰) = 95%

z⁰ = 1.65

z° = (x° - μ)/σ

1.65 = (x° - 10)/3

x⁰ = 14.95