Answer:
The average rate of change of h(x) in the given interval is 7.
Explanation:
When we want to find the average rate of change of a function f(x), in an interval a < x < b, we just need to calculate:
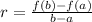
Here we have:
h(x) = 2*x^2 - 7*x
And we want to find the average rate of change between x = 2 and x = 5
This will be:
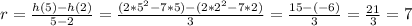
The average rate of change of h(x) in the given interval is 7.