Answer:
The coefficient of the squared term of the equation is 1/9.
Explanation:
We are given that the vertex of the parabola is at (-2, -3). We also know that when the y-value is -2, the x-value is -5. Using this information we want to find the cofficient of the squared term in the parabola's equation.
Since we are given the vertex, we can use the vertex form:
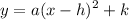
Where a is the leading coefficient and (h, k) is the vertex.
Since the vertex is (-2, -3), h = -2 and k = -3:
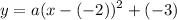
Simplify:

We are also given that y = -2 when x = -5. Substitute:
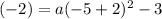
Solve for a. Simplify:

Therefore, our full vertex equation is:
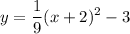
We can expand:
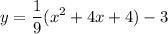
Simplify:
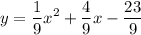
The coefficient of the squared term of the equation is 1/9.