Question

A pizza company runs a marketing campaign based on their delivery time for pizzas. They claim that they will deliver a pizza within 30 minutes of ordering or it is free. In practice the time it takes to prepare a pizza and it being delivered is normally distributed with mean 25 minutes and standard deviation 3 minutes. What is the probability a pizza is delivered for free?On a particular Sunday, 40 pizzas were ordered. What is the probability that more than 2 were delivered for free?If the company wants to reduce the proportion of pizzas that are delivered free to 1%, what should the delivery time be advertised as?

Answer 1

0.0475 = 4.75% probability a pizza is delivered for free.

0.2955 = 29.55% probability that more than 2 were delivered for free.

The delivery time should be advertised as 32 minutes.

Explanation:

To solve this question, we need to understand the binomial distribution and the normal distribution.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Normally distributed with mean 25 minutes and standard deviation 3 minutes.

This means that
`\mu = 25, \sigma = 3`

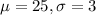
What is the probability a pizza is delivered for free?

More than 30 minutes, which is 1 subtracted by the p-value of Z when X = 30.

`Z = (X - \mu)/(\sigma)`

`Z = (30 - 25)/(3)`

`Z = 1.67`

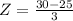
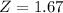
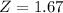`Z = 1.67` has a p-value of 0.9525

1 - 0.9525 = 0.0475

0.0475 = 4.75% probability a pizza is delivered for free

What is the probability that more than 2 were delivered for free?

Multiple pizzas, so the binomial probability distribution is used.

0.0475 probability a pizza is delivered for free, which means that
`p = 0.0475`

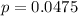
40 pizzas, which means that
`n = 40`

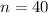
This probability is:

`P(X > 2) = 1 - P(X \leq 2)`

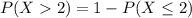
In which

`P(X \leq 2) = P(X = 0) + P(X = 1) + P(X = 2)`

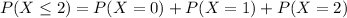
So

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 0) = C_(40,0).(0.0475)^(0).(0.9525)^(40) = 0.1428`

`P(X = 1) = C_(40,1).(0.0475)^(1).(0.9525)^(39) = 0.2848`

`P(X = 2) = C_(40,2).(0.0475)^(2).(0.9525)^(38) = 0.2769`

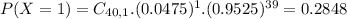
Then

`P(X \leq 2) = P(X = 0) + P(X = 1) + P(X = 2) = 0.1428 + 0.2848 + 0.2769 = 0.7045`

`P(X > 2) = 1 - P(X \leq 2) = 1 - 0.7045 = 0.2955`

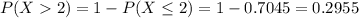
0.2955 = 29.55% probability that more than 2 were delivered for free.

If the company wants to reduce the proportion of pizzas that are delivered free to 1%, what should the delivery time be advertised as?

The 99th percentile, which is X when Z has a p-value of 0.99, so X when Z = 2.327.

`Z = (X - \mu)/(\sigma)`

`2.327 = (X - 25)/(3)`

`X - 25 = 2.327*3`

`X = 32`

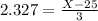
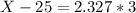
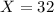
The delivery time should be advertised as 32 minutes.