Question

Which best describes the strength of the model?

O a weak positive correlation
O a strong positive correlation
O a weak negative correlation
O a strong negative correlation

Answer 1

A weak positive correlation

Explanation:

The table of values of the women's age to shoe size is presented as follows;

Women's Age and Shoe Size

`\begin{array}{ccc} Age&&Shoe \, size\\18&&7\\30&&10\\52&&6\\64&&9\end{array}`

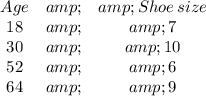
We get;

The correlation coefficient, r, is given as follows;

`r = (\sum \left(x_i - \overline x \right ) \cdot \left(y_i - \overline y \right ))/(√( \sum \left(x_i - \overline x \right )^2 \cdot \sum \left(y_i - \overline y \right )^2))`

From MS Excel, we have;

`\sum \left(x_i - \overline x \right ) \cdot \left(y_i - \overline y \right )` = 2

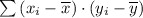`√( \sum \left(x_i - \overline x \right )^2 \cdot \sum \left(y_i - \overline y \right )^2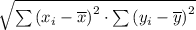)` = √13,000 = 10·√130

∴ r = 2/(10·√130) ≈ 0.01754

Therefore, given that the calculated correlation coefficient, r is positive and less than 0.2 (weak), we have

The correlation is a weak and positive.