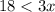Answer:
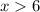
Explanation:
When given the following equation;

One is asked to solve for (
 ). The inequality has exponents, hence, it appears daunting at first, however, the easiest way to deal with exponents is to bring them to the same base. This allows for one to have the ability to treat the exponents like an ordinary number. Since (
). The inequality has exponents, hence, it appears daunting at first, however, the easiest way to deal with exponents is to bring them to the same base. This allows for one to have the ability to treat the exponents like an ordinary number. Since (
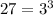 ) one can rewrite (
) one can rewrite (
 ) as (
) as (
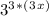 ). This is possible because raising a number to an exponent when it already has an exponent is the same as multiplying the two exponents. Rewrite the inequality in this format;
). This is possible because raising a number to an exponent when it already has an exponent is the same as multiplying the two exponents. Rewrite the inequality in this format;
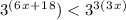
Simplify,
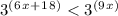
Since the bases are the same they no longer have any relevance, so one can ignore the bases and only work with the exponents,

Now solve this inequality like a normal inequality. Use inverse operations;