Answer:
i. |AB| = 4.70 cm
ii. BAC =

iii. ABC =

Step-by-step explanation:
i Let |AB| be represented by c, |AC| by b and |BC| by a. Since the question would give an included angle triangle, we first apply cosine rule.
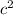 =
=
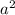 +
+
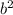 - 2ab Cos C
- 2ab Cos C
=
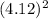 +
+
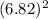 - 2(4.12*6.82) Cos
- 2(4.12*6.82) Cos
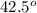
= 16.9744 + 46.5124 - 56.1968*0.7373
= 63.4868 - 41.4340
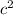 = 22.0528
= 22.0528
c =
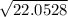
= 4.6960
c = 4.70 cm
Thus |AB| = 4.70 cm
ii. Applying the sine rule;
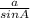 =
=
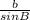 =
=
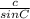
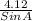 =
=

sin A =
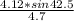
= 0.5922
A =
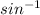 0.5922
0.5922
= 36.3
BAC =

But,
iii. BCA + BAC + ABC =
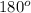
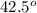 +
+
 + ABC =
+ ABC =
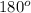
ABC =
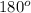 - 78.8
- 78.8
= 101.2
ABC =
