Answer:
14.55 square inches
Explanation:
From the figure it is given that,
The circumference of the top rim of a cone shaped paper cut = 2πr = 7.87 inches
The slant height is l = 3.7 inch
Therefore the least amount of paper which can form a cone shaped cup is the surface area of the cone.
The surface area of the cone is given as :
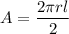
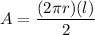
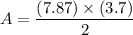
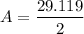
A = 14.55 square inches
Amount of paper required = 14.55 square inches