Answer :
Explanation :
Since the wall with all its bricks makes up the space occupied by it, we need to find the volume of the wall, which is nothing but a cuboid.
Here,
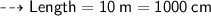
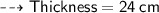
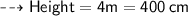
Therefore,

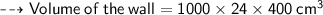
Now, each brick is a cuboid with Length = 24 cm, Breadth = 12 cm and height = 8 cm.
So,
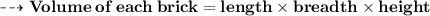

So,
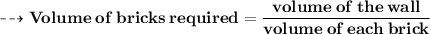


Therefore,
- The wall requires 4167 bricks.