Question

A businessman dropped a coin from the top floor of his office building and it fell according to the formula S(t)=−16t^2+2t^0.5, where t is the time in seconds and S(t) is the distance in feet from the top of the building. Step 1 of 3 : If the coin hit the ground in exactly 1.8 seconds, how high is the building? Round your answer to 2 decimal places.

Answer 1

The height of the building is of 48.24 feet.

Explanation:

Height after t seconds:

The height after t seconds of the coin is given by:

`S(t) = -16t^2 + 2t + h`

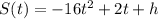
In which h is the height of the building.

If the coin hit the ground in exactly 1.8 seconds, how high is the building?

This means that when
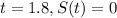`t = 1.8, S(t) = 0`. We use this to find h.

`S(t) = -16t^2 + 2t + h`

`h = 16t^2 - 2t`

`h = 16(1.8)^2 - 2(1.8)`

`h = 48.24`

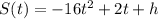
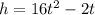
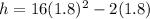
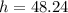
The height of the building is of 48.24 feet.