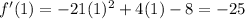Answer:
The slope of the tangent line at x = 1 is -25.
Explanation:
We are given the function:
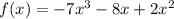
And we want to find the slope of the tangent line at x = 1.
The slope of the tangent line at a point for a function is given by its derivative. Find the derivative of the function:
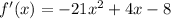
Then the slope of the tangent line at x = 1 is: