Question

Find the equation of a line with a slope of −1/2 that passes through the point −4, 10

Answer 1

Answer:
`y=-(1)/(2) x+8`

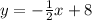
Explanation:

An equation of a line can be in slope intercept form which is y=mx+b

m is the slope, b is the y intercept, x it the x coordinate, and y is the y coordinate. Since we know the slope is -1/2 and we know a x coordinate is -4 and a y coordinate is 10 we can sub them in and solve for the value of b.

`y=mx+b\\10=(-(1)/(2))(-4)+b\\10=2+b\\10-2=2+b-2\\8=b`

The value of b is 8. We can now sub it in for our equation of the line. This time with x and y as variables.

y=-1/2x+8

Answer 2

y - 10 = -1/2(x + 4)

General Formulas and Concepts:

Algebra I

Coordinates (x, y)

Point-Slope Form: y - y₁ = m(x - x₁)

• x₁ - x coordinate
• y₁ - y coordinate
• m - slope

Explanation:

Step 1: Define

Identify variables

m = -1/2

Point (-4, 10) → x₁ = -4, y₁ = 10

Step 2: Find

1. Substitute in variables [Point-Slope Form]: y - 10 = -1/2(x - -4)
2. Simplify: y - 10 = -1/2(x + 4)