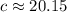Answer:
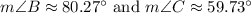
Side AB measures approximately 20.15 units.
Explanation:
We can use the Law of Sines, given by:
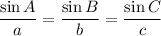
Since we already know ∠A and a, we can use it to determine our other measures.
We know that b is 23. Substitute:
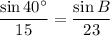
Solve for ∠B:
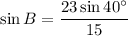
We can take the inverse sine of both sides:
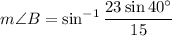
Use a calculator (make sure you're in Degrees mode!):
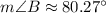
The interior angles of a triangle must total 180°. Hence:

Substitute in known values:
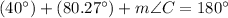
Therefore:

Lastly, to find AB or c, we can use the Law of Cosines:
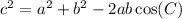
a = 15, b = 23, and to prevent rounding errors, we will use the exact value for C. Recall that we know that:

Using the exact value for ∠B, we acquire:
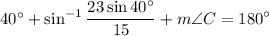
Therefore:
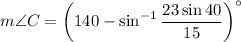
Substitute:
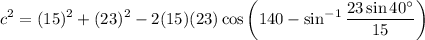
Simplify and take the square root of both sides.
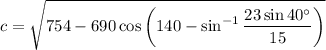
And use a calculator: