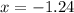Question

Solve the quadratic equation by using a graphic approach. Round your answer to the hundredths place.

x² - 2x - 4= 0
a. x= 3.24 or x = -1.24
c. x = 4.24 or x = -0.24
b. x = 2.73 or x = -0.73
d. x= 5.24or x = -1.24

Pls explain I’m having a hard time understanding the lesson

Answer 1

x = 3.24, x = -1.24

Explanation:

The standard form for a quadratic equation is
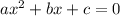`ax^2+bx+c=0`. For your equation a = 1, b = -2, c = -4. The quadratic formula you will be using is
`x=\frac{-b\pm \sqrt{b^(2) -4ac} }{2a}`.

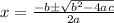Plug in a = 1, b = -2, and c = -4 into the formula.

`=(-\left(-2\right)\pm √(\left(-2\right)^2-4\cdot \:1\cdot \left(-4\right)))/(2\cdot \:1)`

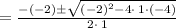
We'll do the top part first:

`√(\left(-2\right)^2-4\cdot \:1\cdot \left(-4\right))`

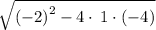
Apply rule
`- (-a) = a`

`=√(\left(-2\right)^2+4\cdot \:1\cdot \:4)`

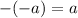
Apply exponent rule
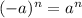`(-a)^(n) =a^n` if
`n` is even

`(-2)^2=2^2`

`=√(2^2+4\cdot \:1\cdot \:4)`

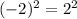
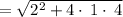
Multiply the numbers

`=√(2^2+16)`

`2^2=4`

`=√(4+16)`

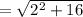
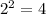
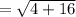
Add

`=√(20)`

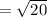
The prime factorization of 20 is
`2^2*5`

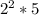
20 divides by 2. 20 = 10 * 2

`=2*10`

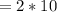
10 divides by 2. 10 = 5 * 2

`=2* \:2*5`

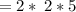
2 & 5 are prime numbers so you don't need to factor them anymore

`=2*2*5`

`=2^2*5`

`=√(2^2\cdot \:5)`

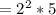
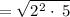
Apply radical rule
`\sqrt[n]{ab} =\sqrt[n]{a} \sqrt[n]{b}`

`=√(5)√(2^2)`

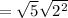
Apply radical rule
`\sqrt[n]{a^(n) } =a`;
`√(2^2) =2`

`=2√(5)`

`=(-\left(-2\right)\pm \:2√(5))/(2\cdot \:1)`

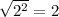
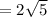
Because of the
`\pm` you have to separate the solutions so that one is positive and the other is negative.

`x=(-\left(-2\right)+2√(5))/(2\cdot \:1),\:x=(-\left(-2\right)-2√(5))/(2\cdot \:1)`

Positive x:

`(-\left(-2\right)+2√(5))/(2\cdot \:1)`

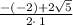
Apply rule
`-(-a)=a`

`=(2+2√(5))/(2\cdot \:1)`

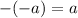
Multiply

`=(2+2√(5))/(2)`

Factor
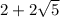`2+2√(5)` and rewrite it as
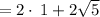`=2\cdot \:1+2√(5)`. Factor out 2 because it is the common term.
`=2\left(1+√(5)\right)`.

`=(2\left(1+√(5)\right))/(2)`

Divide 2 by 2

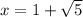`x=1+√(5)` or
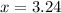`x=3.24` (You'll probably have to use a calculator for the square root of 5)

^Repeating the process of positive x for negative x in order to get
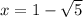`x=1-√(5)` or
`x=-1.24`