Question

How can I factor 5x^2 + 26x - 24 = 0 using the completing the square method.

Answer 1

The answer is -8 with using the completing the square method

Answer 2

QUADRATIC EQUATION

`\mathbb{ANSWER:}`

• 
  `\bold{x = 0.8 \: } \: \sf \: {\color{grey}or} \: \: \: \bold{ x= (4)/(5) } \\`

• 
  `\bold{x = - 6}`

— — — — — — — — — —

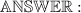
Explanation:

How can we factor 5x^2 + 26x - 24 = 0 using the completing the square method?

Let's solve your equation step-by-step.

`\bold{Given \: Equation: \color{brown} 5x²+26x-24=0}`

First, add 24 to both sides.

• 
  `\bold{5x²+26x-24 - \purple{ 24} = 0 + \purple{24}}`

• 
  `\bold{ \implies \: 5x²+26x = 24 }`

Since the coefficient of 5x² is 5, divide both sides by 5.

• 
  `\bold{ \frac{5 {x}^(2) + 26x }{5} = (24)/(5) } \\`

• 
  `\bold{ \implies \: {x}^(2) + (26)/(5) x = (24)/(5) } \\`

The coefficient of 26/5x is 26/5. So, let b=26/5.

Then we need to add (b/2)²=169/25 to both sides to complete the square.

Add 169/25 to both sides.

• 
  `\bold{ {x}^(2) + (26)/(5) x + \frac{ \purple{169}}{ \purple{25}}= (24)/(5) + \frac{ \purple{169}}{ \purple{25}} } \\`

• 
  `\bold{ \implies \:\bold{ {x}^(2) + (26)/(5) x + \frac{ 169}{ {25}}= (289)/(25) } } \\`

Factor the left side.

• 
  `\bold{(x + (13)/(5) ) {}^(2) = (289)/(25) } \\`

Take square root.

• 
  `\bold{x + (13)/(5) = ± \: \sqrt{ (289)/(25) }} \\`

Then, add (-13)/5 to both sides.

• 
  `\bold{x + (13)/(5) + \frac{\purple{ - 13} }{\purple{ 5}} = \frac{{ - 13} }{{ 5}} ± \: \sqrt{ (289)/(25)}} \\`

• 
  `\bold{ \: x = ( - 13)/(5) ± \sqrt{ (289)/(25) } } \\`

• 
  `\implies \: \underline{ \boxed{ \bold{ (4)/(5) } \sf \: \: or \: \: \bold{ x = - 6}}} \\`

_______________❖_______________