Question

[tex]\sqrt{9-4\sqrt{5} }\:+\sqrt{5}

The answer is 2. Solve if you can. It is 100% correct.

Answer 1

`\\ \rm\Rrightarrow \sqrt{9-4√(5)}+√(5)`

`\\ \rm\Rrightarrow √(9-8.8)+2.2`

`\\ \rm\Rrightarrow √(0.2)+2.2`

`\\ \rm\Rrightarrow 0.3+2.2`

`\\ \rm\Rrightarrow 2.5`

`\\ \rm\Rrightarrow 2(approx)`

Answer 2

Algebraic "proof" that the solution is 2:

`\sqrt{9-4√(5) }\:+√(5)`

`=\sqrt{4-4√(5)+5 }\:+√(5)`

`=\sqrt{2^2-4√(5)+(√(5))^2 }\:+√(5)`

`=\sqrt{(2-√(5))^2 }\:+√(5)`

`=(2-√(5))+√(5)`

`=2`

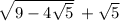
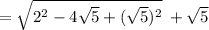
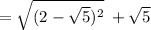
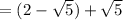
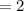
However, this is not the correct mathematical solution to the problem.

The order of operations for
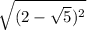`\sqrt{(2-√(5))^2 }` dictate that the operation inside the parentheses must be carried out first.

As
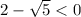`2-√(5) < 0`, then
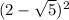`(2-√(5))^2` will always be positive.

If
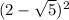`(2-√(5))^2` is always positive, then
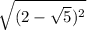`\sqrt{(2-√(5))^2 }` will always be positive.

As √5 > 2 and
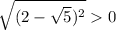`\sqrt{(2-√(5))^2 } > 0` then
`\sqrt{(2-√(5))^2 }\:+√(5) > 2`

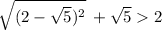
So mathematically, the actual solution to the expression is 2.47 (nearest hundredth) not 2.