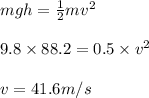Question

You wish to make a simple amusement park ride in which a steel-wheeled roller-coaster car travels down one long slope, where rolling friction is negligible, and later slows to a stop through kinetic friction between the roller coaster's locked wheels sliding along a horizontal plastic (polystyrene) track. Assume the roller-coaster car (filled with passengers) has a mass of 756.5 kg and starts 88.2 m above the ground. (a) Calculate how fast the car is going when it reaches the bottom of the hill.

Answer 1

The speed of roller coaster at the ground is 41.6 m/s.

Step-by-step explanation:

mass of roller coaster, m = 756.5 kg

height, h = 88.2 m

(a) Let the speed of car at the ground is v.

Use conservation of energy

Potential energy at height = kinetic energy at bottom

`m gh = (1)/(2)mv^2\\\\9.8* 88.2= 0.5* v^2\\\\v = 41.6 m/s`