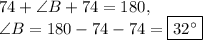Question

What is the measure of <B, in degrees. ​

Answer 1

`\text{A. }32^(\circ)`

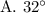
Explanation:

From the Isosceles Base Theorem, the two base angles of a isosceles triangle are equal. Therefore,
`\angle A=\angle C=74^(\circ)`.

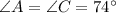Since the sum of the interior angles of a triangle is always equal to 180 degrees, we can write the following equation:

`\angle A+\angle B+\angle C=180`

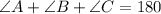
Substitute
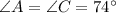`\angle A=\angle C=74^(\circ)` into this equation to solve for
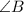`\angle B`:

`74+\angle B+74=180,\\\angle B=180-74-74=\boxed{32^(\circ)}`

Answer 2

32

Explanation:

The base angles of the triangle are the same since the sides are the same

<C = 74

A+B+C = 180 since the sum of the angles of a triangle = 180

74+B+74=180

Combine like terms

148+B =180

B = 180-148

B = 32