Answer:
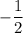
Explanation:
we would like to compute the following limit:
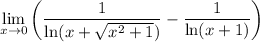
if we substitute 0 directly we would end up with:

which is an indeterminate form! therefore we need an alternate way to compute the limit to do so simplify the expression and that yields:
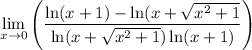
now notice that after simplifying we ended up with a rational expression in that case to compute the limit we can consider using L'hopital rule which states that
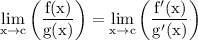
thus apply L'hopital rule which yields:
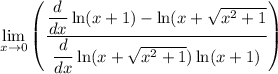
use difference and Product derivation rule to differentiate the numerator and the denominator respectively which yields:

simplify which yields:
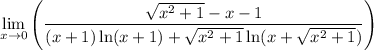
unfortunately! it's still an indeterminate form if we substitute 0 for x therefore apply L'hopital rule once again which yields:
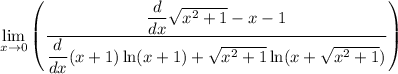
use difference and sum derivation rule to differentiate the numerator and the denominator respectively and that is yields:
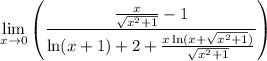
thank god! now it's not an indeterminate form if we substitute 0 for x thus do so which yields:
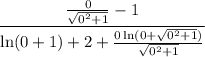
simplify which yields:
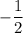
finally, we are done!