Question

In the right triangle AB, mc=90, a=4, and sinA=1/2. what is the length of the hypotenuse?

Answer 1

Given:

In a right angle triangle ABC,
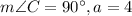`m\angle C=90^\circ , a=4` and
`\sin A=(1)/(2)`.

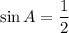To find:

The length of the hypotenuse.

Solution:

It is given that
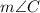`m\angle C`, so opposite side of this angle is the hypotenuse, i.e., c.

In a right angle triangle,

`\sin \theta=(Perpendicular)/(Hypotenuse)`

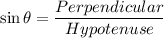
In the given triangle,

`\sin A=(a)/(c)`

Substituting the given values, we get

`(1)/(2)=(4)/(c)`

By cross multiplication, we get

`1* c=4* 2`

`c=8`

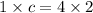
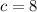
Therefore, the length of the hypotenuse is 8 units.