The question is incomplete. The complete question is :
The pressure difference, Δp, ac
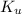 ross a partial blockage in an artery (called a stenosis) is approximated by the equation :
ross a partial blockage in an artery (called a stenosis) is approximated by the equation :

Where V is the blood velocity, μ the blood viscosity {FT/L2}, ρ the blood density {M/L3}, D the artery diameter,
 the area of the unobstructed artery, and A1 the area of the stenosis. Determine the dimensions of the constants
the area of the unobstructed artery, and A1 the area of the stenosis. Determine the dimensions of the constants
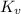 and
and
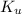 . Would this equation be valid in any system of units?
. Would this equation be valid in any system of units?
Solution :
From the dimension homogeneity, we require :

Here, x means dimension of x. i.e.
![$[ML^(-1)T^(-2)]=([K_v][ML^(-1)T^(-1)][LT^(-1)])/([L])+[K_u][1][ML^(-3)][L^2T^(-2)]$](https://img.qammunity.org/2022/formulas/physics/high-school/xkk8943v89r2i8f9rlt0gvk84ct5hhndfq.png)
![$=[K_v][ML^(-1)T^(-2)]+[K_u][ML^(-1)T^(-2)]$](https://img.qammunity.org/2022/formulas/physics/high-school/e49xkp5ttkdz7e4hn205wadf4etk37qumt.png)
So,
![$[K_u]=[K_v]=[1 ]=$](https://img.qammunity.org/2022/formulas/physics/high-school/bjr1avhme24q93gs4w05mejgqv2d8bu38g.png) dimensionless
dimensionless
So,
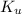 and
and
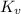 are dimensionless constants.
are dimensionless constants.
This equation will be working in any system of units. The constants
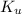 and
and
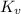 will be different for different system of units.
will be different for different system of units.