Question

What sample size is needed to give a margin of error within in estimating a population mean with 95% confidence, assuming a previous sample had .

Answer 1

`n = ((1.96√(\pi(1-\pi)))/(M))^2`

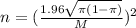
The sample size needed is n(if a decimal number, round up to the next integer), considering the estimate of the proportion
`\pi`(if no previous estimate use 0.5) and M is the desired margin of error.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the z-score that has a p-value of
`1 - (\alpha)/(2)`.

The margin of error is of:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

95% confidence level

So
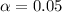`\alpha = 0.05`, z is the value of Z that has a p-value of
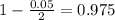`1 - (0.05)/(2) = 0.975`, so
`Z = 1.96`.

Needed sample size:

The needed sample size is n. We have that:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`M = 1.96\sqrt{(\pi(1-\pi))/(n)}`

`√(n)M = 1.96√(\pi(1-\pi))`

`√(n) = (1.96√(\pi(1-\pi)))/(M)`

`(√(n))^2 = ((1.96√(\pi(1-\pi)))/(M))^2`

`n = ((1.96√(\pi(1-\pi)))/(M))^2`

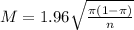
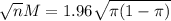
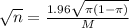
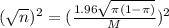
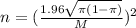
The sample size needed is n(if a decimal number, round up to the next integer), considering the estimate of the proportion
`\pi`(if no previous estimate use 0.5) and M is the desired margin of error.