Question

Suppose that the distance of fly balls hit to the outfield (in baseball) is normally distributed with a mean of 247 feet and a standard deviation of 41 feet. Use your graphing calculator to answer the following questions. Write your answers in percent form. Round your answers to the nearest tenth of a percent. a) If one fly ball is randomly chosen from this distribution, what is the probability that this ball traveled fewer than 216 feet

Answer 1

77.5% probability that this ball traveled fewer than 216 feet.

Explanation:

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Mean of 247 feet and a standard deviation of 41 feet.

This means that
`\mu = 247, \sigma = 41`

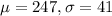
What is the probability that this ball traveled fewer than 216 feet?

The probability as a decimal is the p-value of Z when X = 216. So

`Z = (X - \mu)/(\sigma)`

`Z = (216 - 247)/(41)`

`Z = 0.756`

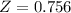
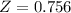`Z = 0.756` has a p-value of 0.775

0.775*100% = 77.5%

77.5% probability that this ball traveled fewer than 216 feet.