Question

A sled with mass 11.00 kg moves in a straight line on a frictionless horizontal surface. At one point in its path, its speed is 4.00 m/s; after it has traveled a distance 3.00 m beyond this point, its speed is 7.00 m/s. Use the work-energy theorem to find the force acting on the sled, assuming that this force is constant and that it acts in the direction of the sled's motion.

Answer 1

The magnitude of the force acting on the sled is 60.5 newtons.

Step-by-step explanation:

The Work-Energy Theorem states that the work done by the external force applied on the sled (
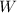`W`), in joules, is equal to the change of its translational kinetic energy (
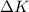`\Delta K`), in joules:

`W = \Delta K` (1)

By definitions of work and translational kinetic energy we expand the equation above:

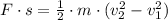`F\cdot s = (1)/(2)\cdot m\cdot (v_(2)^(2)-v_(1)^(2))` (1b)

Where:

`F` - External force applied on the sled, in newtons.

`s` - Travelled distance, in meters.

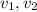`v_(1), v_(2)` - Initial and final velocities, in meters per second.

If we know that
`m = 11\,kg`,
`v_(1) = 4\,(m)/(s)`,
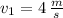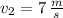`v_(2) = 7\,(m)/(s)` and
`s = 3\,m`, then the external force applied on the sled is:

`F = (m\cdot (v_(2)^(2)-v_(1)^(2)))/(2\cdot s)`

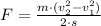
`F = ((11\,kg)\cdot \left[\left(7\,(m)/(s) \right)^(2)-\left(4\,(m)/(s) \right)^(2)\right])/(2\cdot (3\,m))`

`F = 60.5\,N`

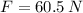
The magnitude of the force acting on the sled is 60.5 newtons.