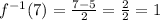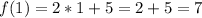Answer:
See explanation
Explanation:
The question has conflicting details
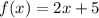
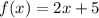 and three halves doesn't sound correct.
and three halves doesn't sound correct.
So, I will take f(x) as
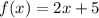
Next, solve for the inverse function
Replace f(x) with y
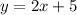
Swap x and y
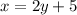
Make 2y the subject
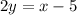
Make y the subject
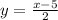
Replace y with the inverse sign
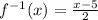
So, now we can calculate any value from the original function and from the inverse function.
For instance: